Light Doesn't Die
Inverse Square Law
If you have spent time around photography, then you have likely heard the phrase “Inverse Square Law” pertaining to lighting. What this notion suggests is that the amount of light coming from a light source becomes a quarter as intense when you double distance from the source, but this isn’t really entirely accurate. This notion oftentimes seems to suggest that light dies, and it doesn’t. The reason the light becomes less ‘intense’ is because the density decreases, not because the light particles diminish in power.
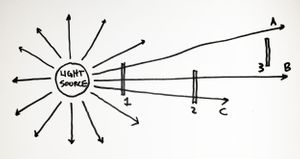
In this example, the amount of light seen at point 1 is greater than at point 2 which is greater than at point 3 because more light particles are intersecting the capture surface, not because any light particles are becoming weaker. Light particle alpha is exactly the same at point 1 as it is at point 3, and in a perfect environment, like a vacuum (like space is), that particle will theoretically travel forever. When we see another galaxy, for example, it is because tiny little light particles (no different from any other) have traveled zillions of light years through space. Light doesn’t travel forever in our atmosphere because the air is full of stuff (water vapor, dust, etc.) that light particles become absorbed or refracted by .
Why Does This Matter?
The reason for why any of this matters to photography lies in looking at the formal definition of the Inverse Square Law:
“The intensity (or illuminance or irradiance) of light or other linear waves radiating from a point source (energy per unit of area perpendicular to the source) is inversely proportional to the square of the distance from the source” (source: Wikipedia).
The key word in there is “point”. The Inverse Square Law assumes light emitting from a point. Light emitting from a point is emanating out in all directions, but photographers don’t always work with point sources. While modifiers like softboxes functionally are close enough to being point sources, what happens when a grid is added or a photographer uses a snoot.
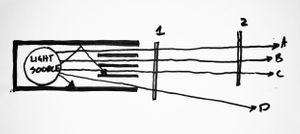
The grid or snoot changes the light from being a point source to being a directional source. Because the only light coming from the modifier is going to tend to be heading in the same direction, light density, intensity, falloff (or whatever) term you want to use, will not be as significant as from a point source, and this light will not follow the inverse square law. Of course, the light coming from the modifier is never going to be perfectly parallel, so there is still going to be light falloff.
Another perfect example of a directional source, of course, is the sun. Light particles from the sun are extremely close to being parallel, so there is no light fall-off at all. If we take a picture of someone in the sun, the light hitting the ground behind them is just as intense as the light hitting them.
Impact in practice?
Honestly, probably not much. Most grids are relativelty shallow and so there will still be plenty of diverging light and therefore these lights will be close to following the Inverse Square Law. Unless a photographer is specifically working with a modifier that is designed for focusing light (like a parabolic reflector or fresnel lens), than it probably doesn't have much of an impact.
